How to Find Complementary and Supplementary Angles? (+FREE Worksheet!)

Complementary and supplementary angles, Example 2:

Complementary and supplementary angles, Example 3:

Two angles are complementary when they add to 90 degrees and supplementary when they add to 180. Angles on a straight line are supplementary; the two acute angles in a right triangle are complementary. Below: how to find the missing angle in each case, with worked examples and a free worksheet.

Tutor-style math help

Supplementary, Complementary, and Vertical Angles: what to notice and how to work it

Geometry skill

Angle relationships let you solve unknown angles without measuring. The key is knowing which total the relationship creates.

What to notice first

Complementary angles total 90 degrees, supplementary angles total 180 degrees, and vertical angles are equal.

Common student mistake

Do not use 90 degrees for every angle pair. Supplementary and linear-pair situations use 180 degrees.

Key formulas and cues

\(\text{complementary: }a+b=90^\circ\)

\(\text{supplementary: }a+b=180^\circ\)

\(\text{vertical angles are equal}\)

A reliable path

Label the diagramWrite each given measurement on the figure.
Choose the formulaMatch the formula to distance, midpoint, area, volume, or angle relationships.
Check unitsUse linear, square, or cubic units as appropriate.

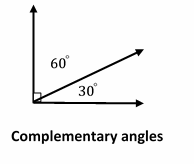
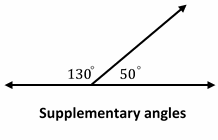
Worked examples

Complementary angles

Example: One angle is \(37^\circ\). Find its complement.

Complements total 90 degrees.
Subtract 37 from 90.
Label the angle.

Answer: \(53^\circ\)

Supplementary angles

Example: One angle is \(118^\circ\). Find its supplement.

Supplements total 180 degrees.
Subtract 118 from 180.
Label the angle.

Answer: \(62^\circ\)

Open pop-up practice

Try one before moving on

Try: Find the supplement of \(73^\circ\).

Answer: \(107^\circ\).

Next step: do the matching worksheet or quiz while the method is still fresh, then come back and explain the first step in your own words.

Complementary and supplementary angles, Example 4:

Find the missing angle.

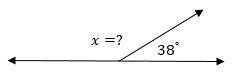
Solution:

Notice that two angles form a straight angle when together. This means that the angles are supplementary and have a sum of \(180^\circ\).
\(x+38=180→x=180-38=142^\circ\)

Exercises for Complementary and Supplementary Angles

Find the missing measurement in the pair of angles.

1. \(\color{blue}{x=}\)

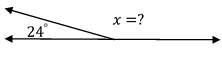
2.\(\color{blue}{x=}\)

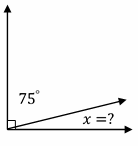
3.\(\color{blue}{x=}\)

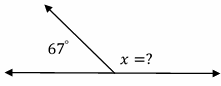
4.\(\color{blue}{x=}\)

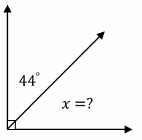
5. The measure of an angle is \(31^°\). What is the measure of its complementary angle? __________

6. The measure of an angle is \(76^°\). What is the measure of its supplementary angle? __________

\(\color{blue}{156^\circ}\)
\(\color{blue}{15^\circ}\)
\(\color{blue}{113^\circ}\)
\(\color{blue}{46^\circ}\)
\(\color{blue}{59^\circ}\)
\(\color{blue}{104^\circ}\)

The Ultimate Algebra Bundle 2026: From Pre-Algebra to Algebra II

Related to This Article

Complementary and supplementary angles

What people say about "Complementary and Supplementary Angles"?

No one replied yet.

How to Find Complementary and Supplementary Angles? (+FREE Worksheet!)

Complementary and supplementary angles, Example 2:

Complementary and supplementary angles, Example 3: