How to Identify the Function from the Graph

Step-by-step Guide to Identify the Function from the Graph

Here is a step-by-step guide to identify the function from the graph:

Step 1: Foundational Grounding

• Familiarize yourself with the basic definition of a function. Recall that a function assigns to every input exactly one output.

Step 2: Utilize the Vertical Line Test

• The vertical line test is a fundamental tool for identifying functions. Draw or imagine vertical lines across the graph:
  • If any vertical line intersects the graph at more than one point, the graph doesn’t represent a function.
  • If every vertical line intersects the graph at most once, it is a function.

Step 3: Spot Special Cases

• Some graphs, like those of vertical lines (e.g., \(x=3\)), will fail the vertical line test immediately. These are never functions.

Step 4: Analyze Continuous vs. Discontinuous Graphs

• Continuous graphs have no breaks, jumps, or holes. If such a graph passes the vertical line test, it represents a continuous function.
• Discontinuous graphs may have gaps or jumps. Each separate piece of the graph should be individually subjected to the vertical line test.

Step 5: Evaluate for Implicit Functions

• Some functions, especially those involving circles or ellipses, might not be explicitly solved for one variable. Even if they appear complex, the vertical line test remains a valid tool.

Step 6: Understand Graph Behavior at Asymptotes

• Vertical asymptotes can be tricky. A graph might approach but never cross a vertical asymptote, which can still be consistent with a function. However, if it crosses an asymptote more than once, it fails the function test.

Step 7: Investigate End Behavior and Horizontal Asymptotes

• While the horizontal asymptotes and end behavior don’t directly determine if a graph is a function, they can give insights into the function’s type and characteristics.

Step 8: Distinguish One-to-One Functions with the Horizontal Line Test

• While the vertical line test determines if a graph is a function, the horizontal line test determines if it’s a one-to-one function. If no horizontal line intersects the graph more than once, the function is one-to-one.

Step 9: Employ Graphing Tools and Software

• Utilize tools like Desmos, GeoGebra, or graphing calculators to visualize and manipulate graphs. These can help in understanding complex graphs and verifying if they represent functions.

Step 10: Engage in Comparative Analysis

• Compare the given graph with standard function shapes you know (e.g., linear, quadratic, sine, exponential). Recognizing similarities or patterns can aid in identification.

Step 11: Iterative Verification

• Continuously re-evaluate as you analyze. Sometimes, initial perspectives might not capture all the nuances of a complex graph.

Step 12: Seek Peer Reviews

• Sometimes, discussing with peers or presenting your analysis to others can help spot oversights or provide alternative viewpoints.

By systematically following this multifaceted approach, one can accurately identify whether a given graph, even one with high variation and complexity, represents a function. This process not only focuses on identification but also deepens one’s understanding of the nature and behavior of functions in general.

Examples:

Given the following graph, determine whether the graph is a function or not.

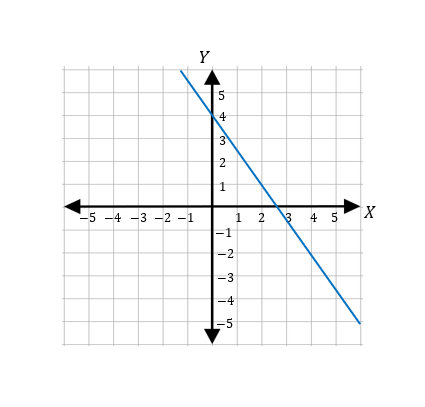
Solution:

Draw a vertical line across the graph such as the line drawn in the graph below. It intersects the graph at most once, So, it is a function.