How to Graph the Tangent Function?

Step-by-step guide to graphing the tangent function

The tangent function is one of the six main trigonometric functions and is generally written as \(tan x\). It is the ratio of the opposite side and the adjacent side of the given angle in a right triangle.

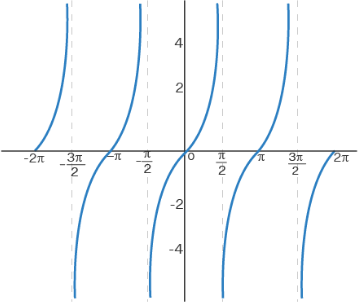
The graph of the \(tan\) function is a discontinuous graph because \(tan x\) is not defined at odd multiples of \(\frac{π}{2}\), that is, \(tan x\) is not defined for \(x = \frac{kπ}{2}\), where \(k\) is an odd integer. Also, since the \(tan\) function has a period of \(π\), its values repeat after every \(π\) radian, and hence, the curve pattern repeats after every \(π\) radian. As we can see in the graph of the tangent function given below, the function has vertical asymptotes at \(x=-\frac{\pi \:\:}{2},\:\frac{\pi \:\:}{2},\:-\frac{3\pi \:\:}{2},\:\frac{3\pi \:\:}{2},\:\frac{5\pi \:\:}{2},…\).