How to Solve and Graph One-Step Multiplication and Division Equations
This step-by-step guide for solving One-Step equations will teach you to solve and graph one-step multiplication and division equations with sample questions and solutions.

Solving one-step equations is an important math skill to help students find the value of a variable that makes an equation true.
For example, consider the equation 4x = 12. To solve for x, you only need to divide both sides of the equation by 4, which gives you x = 3.
Steps to Solving & Graphing One-Step Multiplication and Division Equations
One-Step equations are ones that merely require one math operation to be solved.
You can use these four rules to solve one-step equations:
- Both sides stay equal if you add the same number to both sides of an equation.
- Both sides stay equal if you subtract the same number from both sides of an equation.
- Both sides stay equal if you divide both sides of an equation by the same number.
- Both sides stay equal if you multiply both sides of an equation by the same number.
Here is a step-by-step guide to solving one-step multiplication and division equations:
Step 1: Identify the variable and the operation
Determine which variable you are trying to solve for and identify whether the operation in the equation is addition, subtraction, multiplication, or division.
Step 2: Isolate the variable
Use inverse operations to isolate the variable on one side of the equation. If the operation is multiplication, divide both sides of the equation by the constant. If the operation is divided, multiply both sides of the equation by the constant.
Step 3: Check your solution
Plug in your solution to the original equation to ensure it is correct.
Sample Questions of One-Step Multiplication and Division Equations
Examples 1 – solve the equation and graph the solution
Solve the equation. And graph the solution, \(\frac{x}{2}=5\)
Solution
Use inverse operations for \(x\) to solve the equation.
Since x is divided by 2 in this equation, its inverse is multiplied by 2 on both sides. \(\frac{x}{2}×2=5×2→x=10\). Now, graph 10 on the number line.
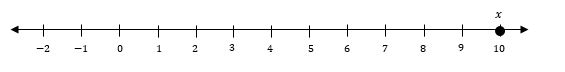
Examples 2 – solve the equation and graph the solution
Solve the equation. And graph the solution \(9v=27\)
Solution
Use inverse operations for v to solve the equation.
Since v is multiplied by 9 in this equation, then its inverse is divided by 9 into both sides, \(\frac{9v}{9}=\frac{27}{9}→x=3\). Now, graph \(x=3\) on the number line.
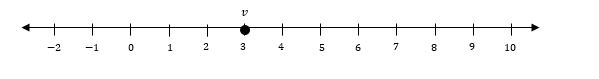
Related to This Article
More math articles
- 7th Grade Georgia Milestones Assessment System Math FREE Sample Practice Questions
- 7th Grade NHSAS Math Worksheets: FREE & Printable
- The Ultimate College Mathematics Placement Course (+FREE Worksheets & Tests)
- 6th Grade Scantron Math Worksheets: FREE & Printable
- 10 Most Common 6th Grade SBAC Math Questions
- FREE 5th Grade ACT Aspire Math Practice Test
- How to Find Fractions of Time Units
- How to use Intercepts
- 10 Most Common 6th Grade MCAS Math Questions
- Full-Length 7th Grade PSSA Math Practice Test





























What people say about "How to Solve and Graph One-Step Multiplication and Division Equations - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.