How to Solve and Graph One-Step Inequalities with Rational Numbers?
In this step-by-step guide, you will learn how to solve and graph one-step inequalities with rational numbers.

A step-by-step guide to solving and graphing one-step inequalities with rational number
To graph an inequality on a number line, you have to pick between using a filled-in or open circle.
Whenever an inequality represents \(≤\) or \(≥\), utilize a filled-in circle.
Whenever an inequality represents \(<\) or \(>\), utilize an open circle.
To solve the problems, you must utilize inverse operations.
Here’s a step-by-step guide to solving and graphing one-step inequalities with rational numbers:
Step 1: Understand the inequality
Before you begin solving the inequality, make sure you understand what it means. Inequalities are expressions that show a relationship between two quantities that are not equal. For example, the inequality \(x + 3 < 7\) means that \(x + 3\) is less than \(7\).
Step 2: Isolate the variable
To solve the inequality, you need to isolate the variable on one side of the inequality symbol. To do this, you can use inverse operations. For example, if the inequality is \(\frac{x}{2} > 5\), you can isolate \(x\) by multiplying both sides of the inequality by \(2\), which gives you \(x > 10\).
Step 3: Check the solution
Once you’ve isolated the variable, you should check your solution to make sure it’s valid. To do this, substitute your solution back into the original inequality and see if it makes sense. For example, if the inequality is \(\frac{x}{2} > 5\) and you solved for \(x = 12\), you can check by substituting \(12\) back into the inequality: \(\frac{12}{2} > 5\), which is true, so the solution is valid.
Step 4: Graph the solution
To graph the solution, you can use a number line. First, plot the solution as a dot on the number line. Then, shade in the appropriate region of the number line based on whether the inequality is less than, greater than, less than, equal to, or greater than or equal to. For example, if the inequality is \(x > 3\), you would plot a dot on \(3\) and shade in the region to the right of \(3\).
Step 5: Write the solution in interval notation
Finally, you can write the solution in interval notation. This notation uses brackets and parentheses to indicate whether the endpoints of the interval are included or excluded. For example, if the solution is \(x > 3\), you would write it as (\(3\), infinity), where the parentheses around \(3\) indicate that it is not included in the interval, and the infinity symbol indicates that the interval extends indefinitely to the right.
Solving and Graphing One-Step Inequalities with Rational Numbers – Examples 1
Solve the inequality \(2w≥10\), then graph on the number line.
Solution:
Use inverse operations for w to solve the problem. Since w is multiplied by \(2\), then its inverse is divided by \(2\) into both sides, \(\frac{2w}{2}=\frac{10}{2}→w≥5\). The \(w≥5\) inequality means any number greater than or equal to \(5\), so you must utilize a filled-in circle.

Solving and Graphing One-Step Inequalities with Rational Numbers – Examples 2
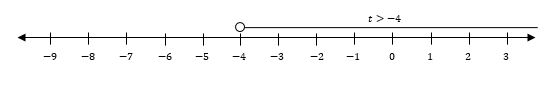
Solve the inequality \(\frac{t}{4}>-1\), then graph on the number line.
Solution:
Use inverse operations for \(t\) to solve the problem. Since t is divided by \(4\) in this inequality, then its inverse is multiplied by \(4\) on both sides to find the solution, \(\frac{t}{4}×4>-1×4→t>-4\). The \(t>-4\) inequality means any number greater than \(t>-4\), so you must utilize an open circle.
Related to This Article
More math articles
- How to Decipher Patterns: A Comprehensive Guide to Understanding Mathematical Sequences
- How To Choose the Right DAT Course?
- Quotient Quickies: How to Navigate Decimal Division with Estimations
- 4th Grade K-PREP Math Worksheets: FREE & Printable
- TABE Math Formulas
- How to Use Place Value Blocks to Compare Decimals
- 8th Grade NJSLA Math Worksheets: FREE & Printable
- Top 10 SAT Math Prep Books (Our 2023 Favorite Picks)
- The Ultimate 7th Grade Scantron Math Course (+FREE Worksheets)
- FREE 6th Grade ACT Aspire Math Practice Test


























What people say about "How to Solve and Graph One-Step Inequalities with Rational Numbers? - Effortless Math: We Help Students Learn to LOVE Mathematics"?
No one replied yet.